What factors can affect the optimal design of F-shaped traffic signposts
Traffic Signs is to guide road users to comply Traffic order, making road traffic safe, efficient, and smooth running infrastructure. In order to make the traffic signs meet the requirements of use and maintain durability in different environments, the mechanical strength, stiffness, stability and steel consumption under load should be fully considered in the design.
In recent years, many scholars at home and abroad have done research on it, using MathCAD software, which greatly simplifies the manual calculation work in traffic sign structure design, and facilitates design. The personnel grasp the entire calculation process, and can quickly modify the template when the modification is needed, which improves the design work efficiency. The technology has carried out a full-scale model test on a new type of socket-type F-shaped traffic sign structure.
The finite element method was used to optimize the traffic sign structure, and the conventional finite element method and the precise finite element method in the design of traffic sign structure were compared and analyzed. The optimal design of this paper is carried out from the dimensions of the components, observing its influence on the F-shaped traffic sign poles, and maximizing the mechanical properties of the materials to reduce the cost. At the same time, the research results can provide a basis for the design of variable cross-section traffic sign structure.
1 Basic Theory
The penalty function, also called the penalty function, is a type of constraint function whose basic idea is to impose a penalty by adding a penalty term after the objective function. The constraint condition is weighted, and the initial objective function is constructed into a new objective function, and the constrained optimization problem is transformed into several unconstrained optimization problems. At the same time, the process is also applicable to the extreme value problem without the constraint extreme value seeking the most advantage. Suppose the objective function is
y = f( x 1 ,x 2 ,...,x n ) = f( X) , ( 1)
where: y is the minimum value.
Under the equality constraint, there is
g j ( X) = 0( j = 1,2,...,m) . (2)
To find a new objective function, then P( X,λ k ) = f( X) + λ k ∑
mj = 1g j ( X [ ] )2 . (3)
As the λ k increases, the constraint constraint is gradually increased. When λ k =0, the constraint can be ignored. When λ k →∞, the function P The minimum value is towards y minimum. When the condition does not reach the standard, the larger the λ k is, the larger the value of the penalty function P is. When the constraint cannot be satisfied, the function P covers the penalty of the objective function, ie
λ k ∑ Mi = 1[gi ( x) ]=0,x ∈ D> 0,x {D. (4)
Under the inequality constraint
min f( x) , x ∈ R n , s. t. g j ( X) ≥ 0, i = 1, 2, ..., m . (5)
Define the penalty function as
P( X,λ k ) = f( X) + λ k ∑mi = 1[min( 0,gi ( x) ) ]2 ,( 6)
where λ k is a sufficiently large positive number, and
λ k ∑mi = 1[min( 0,gi ( x) ) ]
2=0, x ∈ D> 0, x {D,( 7)
minP( X,λ k ) . (8)
This paper takes the penalty function method in the constrained optimization method as the basic theory of optimization design. The research object is the equal-section F-shaped traffic sign structure. The optimization content includes the section size and section form, and the section form includes Variable cross section form. In recent years, finite element has developed rapidly, and its application has been generally fast and derived a new type of optimization design method, namely the section shape optimization technique of finite element software analysis. By providing a conceptual design at the design stage, a reasonable cross-sectional shape layout of the overall model and individual components is obtained, thereby optimizing the overall structure. Not only reduces the application of the material, but also meets the stiffness and strength requirements of the component.
2 Calculation Cases
2.1 Project Situation
This paper selects the square-shaped F-shaped traffic sign structure commonly used in road engineering as the research object, respectively. The size of the beam is divided into three models, as shown in Table 1.
Traffic sign structure The material is Q235. Its flexural, compressive and tensile strength design values and standard yield stress are both 215 MPa, the shear strength design value is 125 MPa, the elastic modulus is 206 GPa, and the shear modulus is 79 GPa. The signboard is made of aluminum alloy, the mass per unit area is 8.037 kg/m 2 , and the size of the signboard is 4 000 mm × 2 000 mm (length × width) according to the signboard. Under normal circumstances, under the action of wind load (standard value), the horizontal displacement of any point of the traffic sign structure shall not be greater than 1/75 of the height of the point from the top of the foundation. For the cantilever type sign structure, the relative horizontal displacement of the end of the beam shall not be allowed. More than 1/75 of its length, the total horizontal displacement shall not be greater than 1/50 of the height from the top of the foundation.
2.2 Calculation Model
Because the F-shaped traffic sign bar is a steel structure, under the premise of retaining the main mechanical properties of its steel structure, to avoid the complexity of the problem The model is simplified as follows:
1) Ignore the flange and bolt connections in the calculation model;
2) Assume that the connection between the upper structure of the calculation model and the foundation is just connected, That is to constrain all 6 degrees of freedom at the root of the column.
2.3 Basic Assumptions
1) Loads: Only gravity loads and wind loads are considered, and seismic loads and vehicle loads and vehicle induced gusts are ignored.
2) Flange connection: Since the function of the flange is mainly reflected in transportation and installation, the influence on the mechanical properties of the structure is relatively small, and the flange and bolt connection will be ignored in the calculation model.
3) Signboard load transfer: For the calculation model of this paper, it is assumed that the gravitational load and wind load of the signboard are equivalent to the line load on the beam.
4) Load combination: This paper only considers the load combination under the most unfavorable conditions, that is, the combination of wind load and gravity load under the condition that the wind load is perpendicular to the sign board surface.
3 finite element analysis
The optimal design problem of this paper is to make the traffic sign structure under the conditions of structural bending and shear strength, structural deformation conditions and structural stability. The lightest problem. From the optimization of design variables, constraints and objective functions, the finite element model is established by Ansys.
3.1 Modeling
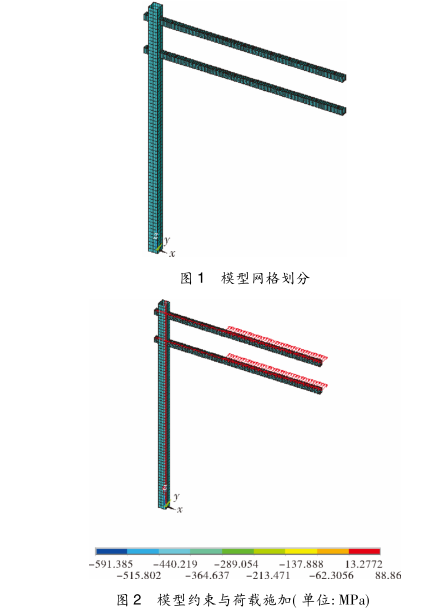
The 3D linear finite strain cell BEAM188 unit is selected for its analysis of slender to medium slender beam structures based on the Timoshenko beam ( Timoshenko) theory, considering the effects of shear deformation. First define the size and position of each node in the space, then load input, and finally set the unit length, divide the grid, and generate the finite element model, as shown in Figure 1-2.
3.2 Optimization Results
The finite element model is established, the internal force calculation and parameter extraction are completed by the above commands, and the optimization analysis file is written by the Lgwrite command.


